• home
• sitemap
• LiveMath info
• Flash highlights
• Scientific Notebook
• math blog
• about
• feedback

What are the frequencies of music notes?

In the table of frequencies below, you'll find A = 440 Hz, and then

A# = 466.16 Hz,
B = 493.88 Hz,
C = 523.25 Hz, etc.

Also, you can find Middle C: 261.63 Hz.

Here is a LiveMath animation which illustrates these frequencies.

LIVEMath

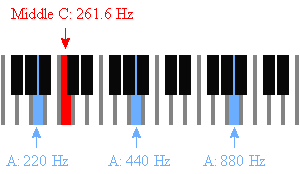
Table of Musical Frequencies

These are found using

frequency = 440×(2^n/12)

for n = -21, -19, ..., 27

Where did this come from?

This problem reminded me of Compound Interest that we met earlier in Money Math. The frequency needs to double every 12 notes (because there are 7 white notes and 5 black notes in each octave.)

Here is a graph of that relationship: frequency = 440×(2^n/12)

This is an exponential curve, that we met earlier in Graphs of Exponential Functions.

Equal Tempered Tuning

An interesting problem has faced musical instrument makers for hundreds of years. To get a "perfect 5th" (the interval between A and the E above, say), we need to play a note which has 1.5 times the frequency of A.

On a violin (or viola or any fretless stringed instrument) this is possible, and we can play a beautiful, perfect E at 440 x 1.5 = 660 Hz. But notice (from the frequency table above) that a piano playing the same note will play E = 659.26 Hz [just a little flat!].

Around 400 years ago, keyboards (usually harpsichords and organs) were tuned for a particular key (say D major), so that all the instruments, especially strings, sounded "right" in that key. The harpsichord sounded great in that key, but pretty awful in other unrelated keys (say B flat).

Around the time of J. S. Bach, it was decided to tune keyboards so that the notes were evenly spaced. Then the keyboard could play in any key more successfully. This is called equal tempered tuning.

Unfortunately, it means all stringed instruments have to allow for the slight differences in tunings between instruments. Strings are usually happiest when playing with other strings only, for this reason.

Return

Chapter Contents

• Graphs of the Trigonometric Functions
• 1. Graphs of y = a sin x and y = a cos x
• 2. Graphs of y = a sin bx and y = a cos bx
• Biorhythm Graphs
• 3. Graphs of y = a sin(bx + c) and y = a cos(bx + c)
• 4. Graphs of tan, cot, sec and csc
• 5. Applications of Trigonometric Graphs
• 6. Composite Trigonometric Graphs
• 7. Lissajous Figures

• Comments, Questions?

♫ Music while you math...

Go ahead, enjoy! ♫

Choose your music ____________ Classical Pop Rock Classic rock Indie rock Singer-songwriter Female vocalist Soundtrack Ambient

Newsletter

From the math blog...